Difference between revisions of "Regularized moduli spaces"
From Polyfolds.org
m |
m |
||
| Line 1: | Line 1: | ||
[[table of contents]] | [[table of contents]] | ||
| − | Given the [[moduli spaces of pseudoholomorphic polygons]] <math>\overline\mathcal{M}(x_0;x_1,\ldots,x_d)</math> for each tuple of Lagrangians <math>L_0,\ldots,L_d\subset M</math>, generators <math>x_0\in\text{Crit}(L_0,L_d), x_1\in\text{Crit}(L_0,L_1), \ldots, x_d\in\text{Crit}(L_{d-1},L_d)</math>, and a fixed compatible almost complex structure <math>J</math>, we need to explain how to obtain regularizations <math>\overline\mathcal{M}^k(x_0;x_1,\ldots,x_d;\nu)</math> for expected dimensions <math>k=0,1</math> by a choice of perturbations <math>\nu</math>. | + | Given the [[moduli spaces of pseudoholomorphic polygons]] <math>\overline\mathcal{M}(x_0;x_1,\ldots,x_d)</math> for each tuple of Lagrangians <math>L_0,\ldots,L_d\subset M</math>, generators <math>x_0\in\text{Crit}(L_0,L_d), x_1\in\text{Crit}(L_0,L_1), \ldots, x_d\in\text{Crit}(L_{d-1},L_d)</math>, and a fixed compatible almost complex structure <math>J</math>, |
| + | |||
| + | |||
| + | talk about Fredholm index <math>\overline\mathcal{M}^k(\ldots) = \{ b\in \mathcal{M}(\ldots) \,|\, IND ... = k\}</math> | ||
| + | |||
| + | |||
| + | we need to explain how to obtain regularizations <math>\overline\mathcal{M}^k(x_0;x_1,\ldots,x_d;\nu)</math> for expected dimensions <math>k=0,1</math> by a choice of perturbations <math>\nu</math>. | ||
Moreover, we need to choose these perturbations ''coherently'' to ensure that the boundary of each 1-dimensional part is given by Cartesian products of 0-dimensional parts, | Moreover, we need to choose these perturbations ''coherently'' to ensure that the boundary of each 1-dimensional part is given by Cartesian products of 0-dimensional parts, | ||
<center> | <center> | ||
Revision as of 22:57, 28 May 2017
Given the moduli spaces of pseudoholomorphic polygons 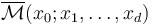 for each tuple of Lagrangians
for each tuple of Lagrangians 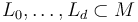 , generators
, generators 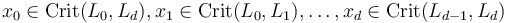 , and a fixed compatible almost complex structure
, and a fixed compatible almost complex structure  ,
,
talk about Fredholm index 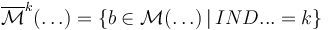
we need to explain how to obtain regularizations 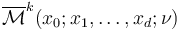 for expected dimensions
for expected dimensions 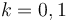 by a choice of perturbations
by a choice of perturbations 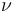 .
Moreover, we need to choose these perturbations coherently to ensure that the boundary of each 1-dimensional part is given by Cartesian products of 0-dimensional parts,
.
Moreover, we need to choose these perturbations coherently to ensure that the boundary of each 1-dimensional part is given by Cartesian products of 0-dimensional parts,

TODO
Finally, we need to check that for each pair 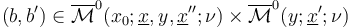 ,
when considered as boundary point
,
when considered as boundary point 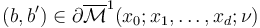 , has symplectic area
, has symplectic area  and weight function
and weight function 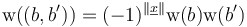 .
.