Difference between revisions of "Global Polyfold Fredholm setup"
From Polyfolds.org
Natebottman (Talk | contribs) |
Natebottman (Talk | contribs) |
||
| Line 1: | Line 1: | ||
== EP-groupoid basics == | == EP-groupoid basics == | ||
| + | |||
| + | A polyfold should be an "M-polyfold with isotropy". | ||
| + | This is implemented via the language of EP-groupoids, which in finite dimensions reduce to orbifolds. | ||
| + | First, recall that a '''groupoid''' is a small category in which every morphism is invertible (hence a groupoid with a single object is the same thing as a group). | ||
| + | Now define an '''EP-groupoid''' to be a groupoid <math>X</math> with morphism set <math>\mathbf X</math> satisfying these properties: | ||
| + | * '''(Lie)''' <math>X</math> and <math>\mathbf X</math> are equipped with M-polyfold structures, with respect to which the source, target, multiplication, unit, and inversion maps | ||
| + | <center><math> | ||
| + | s: \mathbf X \to X, \quad t: \mathbf X \to X, \quad m: \mathbf X {}_s\times_t \mathbf X \to X, \quad u: X \to \mathbf X, \quad i: \mathbf X \to \mathbf X | ||
| + | </math></center> | ||
| + | are sc-smooth. | ||
| + | * '''(etale)''' <math>s</math> and <math>t</math> are surjective local sc-diffeomorphisms. | ||
| + | * '''(proper)''' For every <math>x \in X</math>, there exists a neighborhood <math>V(x)</math> so that <math>t: s^{-1}\bigl(\overline{V(x)}\bigr) \to X</math> is proper. | ||
| + | |||
<div class="toccolours mw-collapsible mw-collapsed"> | <div class="toccolours mw-collapsible mw-collapsed"> | ||
Revision as of 15:31, 2 June 2017
EP-groupoid basics
A polyfold should be an "M-polyfold with isotropy".
This is implemented via the language of EP-groupoids, which in finite dimensions reduce to orbifolds.
First, recall that a groupoid is a small category in which every morphism is invertible (hence a groupoid with a single object is the same thing as a group).
Now define an EP-groupoid to be a groupoid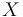 
with morphism set 
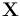satisfying these properties:
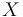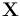- (Lie) and are equipped with M-polyfold structures, with respect to which the source, target, multiplication, unit, and inversion maps
are sc-smooth.
- (etale)
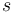and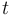 
are surjective local sc-diffeomorphisms. - (proper) For every
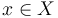, there exists a neighborhood 
so that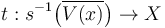 
is proper.
Example: We work out the EP-groupoid structure of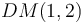 
in detail.
blah blah
