Difference between revisions of "Moduli spaces of pseudoholomorphic polygons"
m |
m (→General moduli space of pseudoholomorphic polygons) |
||
| Line 400: | Line 400: | ||
</math> | </math> | ||
</center> | </center> | ||
| − | which - since <math>\ | + | which - since <math>\omega|_{L_i}\equiv 0</math> only depends on the total homology class of the generalized polygon |
<center> | <center> | ||
<math> | <math> | ||
Revision as of 23:48, 28 May 2017
To construct the moduli spaces from which the composition maps are defined we fix an auxiliary almost complex structure 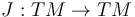 which is compatible with the symplectic structure in the sense that
which is compatible with the symplectic structure in the sense that 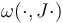 defines a metric on
defines a metric on 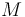 . (Unless otherwise specified, we will use this metric in all following constructions.)
. (Unless otherwise specified, we will use this metric in all following constructions.)
Then given Lagrangians 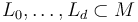 and generators
and generators 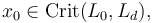

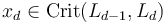 of their morphism spaces, we need to specify the Gromov-compactified moduli space
of their morphism spaces, we need to specify the Gromov-compactified moduli space 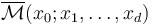 . (Here and throughout, we will call a moduli space Gromov-compact if its subsets of bounded symplectic area are compact in the Gromov topology.)
We will do this by combining two special cases which we discuss first.
. (Here and throughout, we will call a moduli space Gromov-compact if its subsets of bounded symplectic area are compact in the Gromov topology.)
We will do this by combining two special cases which we discuss first.
Contents
Pseudoholomorphic polygons for pairwise transverse Lagrangians
If each consecutive pair of Lagrangians is transverse, 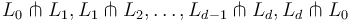 , then our construction is based on pseudoholomorphic polygons
, then our construction is based on pseudoholomorphic polygons

where 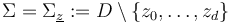 is a disk with
is a disk with 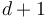 boundary punctures in counter-clockwise order
boundary punctures in counter-clockwise order 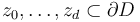 , and
, and 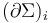 denotes the boundary component between
denotes the boundary component between 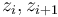 (resp. between
(resp. between  for i=d).
More precisely, we construct the (uncompactified) moduli spaces of pseudoholomorphic polygons for any tuple
for i=d).
More precisely, we construct the (uncompactified) moduli spaces of pseudoholomorphic polygons for any tuple 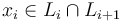 for
for 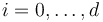 as in [Seidel book]:
as in [Seidel book]:
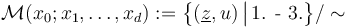
where
-
 is a tuple of pairwise disjoint marked points on the boundary of a disk, in counter-clockwise order.
is a tuple of pairwise disjoint marked points on the boundary of a disk, in counter-clockwise order. -
 is a smooth map satisfying
is a smooth map satisfying
- the Cauchy-Riemann equation
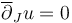 ,
, - Lagrangian boundary conditions
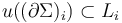 ,
, - the finite energy condition
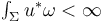 ,
, - the limit conditions
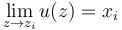 for
for  .
.
- the Cauchy-Riemann equation
- The pseudoholomorphic polygon
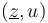 is stable in the sense that the map
is stable in the sense that the map  is nonconstant if the number of marked points is
is nonconstant if the number of marked points is 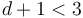 .
.
Here two pseudoholomorphic polygons are equivalent 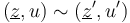 if there is a biholomorphism
if there is a biholomorphism 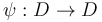 that preserves the marked points
that preserves the marked points 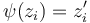 , and relates the pseudoholomorphic polygons by reparametrization,
, and relates the pseudoholomorphic polygons by reparametrization, 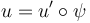 .
.
The case 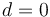 is not considered in this part of the moduli space setup since
is not considered in this part of the moduli space setup since 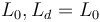 are never transverse. However, it might appear in the construction of homotopy units?
are never transverse. However, it might appear in the construction of homotopy units?
The domains of the pseudoholomorphic polygons are strips for 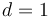 and represent elements in a Deligne-Mumford space for
and represent elements in a Deligne-Mumford space for 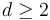 as follows:
as follows:
For 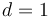 , the twice punctured disks are all biholomorphic to the strip
, the twice punctured disks are all biholomorphic to the strip ![\Sigma _{{\{z_{0},z_{1}\}}}\simeq \mathbb{R} \times [0,1]](/images/math/8/b/3/8b3c9f4dfa661a678858f5301011005f.png) , so that we could equivalently set up the moduli spaces
, so that we could equivalently set up the moduli spaces 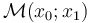 by fixing the domain
by fixing the domain ![\Sigma _{{d=1}}:=\mathbb{R} \times [0,1]](/images/math/b/9/b/b9b8b4c742fe7b67774c1dfe2eb02833.png) and defining the equivalence relation
and defining the equivalence relation 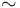 only in terms of the shift action
only in terms of the shift action 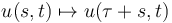 of
of 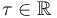 . This is the only case in which the stability condition is nontrivial: It requires the maps
. This is the only case in which the stability condition is nontrivial: It requires the maps ![u:\mathbb{R} \times [0,1]\to M](/images/math/3/9/e/39e5527f65a10854493238103878da42.png) to be nonconstant.
to be nonconstant.
For 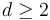 , the moduli space of domains
, the moduli space of domains
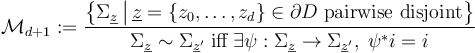
can be compactified to form the Deligne-Mumford space  , whose boundary and corner strata can be represented by trees of polygonal domains
, whose boundary and corner strata can be represented by trees of polygonal domains 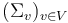 with each edge
with each edge  represented by two punctures
represented by two punctures  and
and 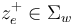 . The thin neighbourhoods of these punctures are biholomorphic to half-strips, and a neighbourhood of a tree of polygonal domains is obtained by gluing the domains together at the pairs of strip-like ends represented by the edges.
. The thin neighbourhoods of these punctures are biholomorphic to half-strips, and a neighbourhood of a tree of polygonal domains is obtained by gluing the domains together at the pairs of strip-like ends represented by the edges.
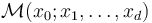 are trivial; that is any disk biholomorphism
are trivial; that is any disk biholomorphism 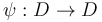 that fixes
that fixes 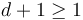 marked points
marked points 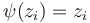 , and preserves a pseudoholomorphic map
, and preserves a pseudoholomorphic map 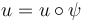 must be the identity
must be the identity 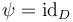 .
.  this follows directly from the marked points, since any Mobius transformation that fixes three points is the identity.
this follows directly from the marked points, since any Mobius transformation that fixes three points is the identity.
In case 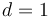 this requires both the stability and finite energy conditions: The group of biholomorphisms that fix two marked points - i.e. the biholomorphisms of the strip - are shifts by
this requires both the stability and finite energy conditions: The group of biholomorphisms that fix two marked points - i.e. the biholomorphisms of the strip - are shifts by 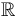 .
On the other hand, any J-holomorphic map
.
On the other hand, any J-holomorphic map ![u:\mathbb{R} \times [0,1]\to M](/images/math/3/9/e/39e5527f65a10854493238103878da42.png) has nonnegative energy density
has nonnegative energy density 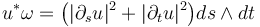 with
with  .
If we now had nontrivial isotropy, i.e.
.
If we now had nontrivial isotropy, i.e. 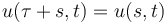 for some
for some 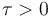 and a nonconstant map
and a nonconstant map 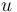 , then there would exist
, then there would exist ![s_{0},t_{0}\in \mathbb{R} \times [0,1]](/images/math/4/a/5/4a585dfca885c55d7af17fbe38e8de85.png) with
with 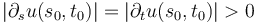 and thus
and thus
![\textstyle \int _{{[s_{0}-{\frac {1}{2}}\tau ]\times [0,1]}}^{{[s_{0}+{\frac {1}{2}}\tau ]\times [0,1]}}u^{*}\omega >0](/images/math/4/c/0/4c0d3fcb1b294a69a84d18c923afba12.png) .
However, this is in contradiction to
.
However, this is in contradiction to 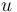 having finite energy,
having finite energy,
![\infty >\textstyle \int _{{\mathbb{R} \times [0,1]}}u^{*}\omega =\sum _{{k\in \mathbb{Z } }}\int _{{[s_{0}+(k-{\frac {1}{2}})\tau ]\times [0,1]}}^{{[s_{0}+(k+{\frac {1}{2}})\tau ]\times [0,1]}}u^{*}\omega =\sum _{{k\in \mathbb{Z } }}\int _{{[s_{0}-{\frac {1}{2}}\tau ]\times [0,1]}}^{{[s_{0}+{\frac {1}{2}}\tau ]\times [0,1]}}u^{*}\omega .](/images/math/5/6/5/56523b116cf68bcb2660fce82a153701.png)
Next, to construct the Gromov-compactified moduli spaces 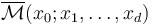 we have to add various strata to the moduli space of pseudoholomorphic polygons without breaking or nodes
we have to add various strata to the moduli space of pseudoholomorphic polygons without breaking or nodes 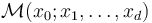 defined above.
defined above.
This is done precisely in the general construction below, but roughly requires to include breaking and bubbling, in particular
- include degenerate pseudoholomorphic polygons given by a tuple of pseudoholomorphic maps
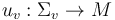 whose domain is a nontrivial tree of domains
whose domain is a nontrivial tree of domains ![[(\Sigma _{v})_{{v\in V}},(z_{e}^{\pm })_{{e\in E}}]\in \overline {\mathcal {M}}_{{d+1}}](/images/math/8/b/6/8b69080d370c68dbb8f70d2174ba64b5.png) ;
; - allow for Floer breaking at each puncture of the domains
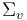 , i.e. a finite string of pseudoholomorphic strips in
, i.e. a finite string of pseudoholomorphic strips in 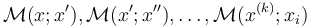 ;
; - allow for disk bubbling at any boundary point of the above domains, i.e. a tree, each of whose vertices is represented by a pseudoholomorphic disk, with edges representing nodes - given by marked points on different disks at which the maps satisfy a matching condition;
- allow for sphere bubbling at any (boundary or interior) point of each of the above domains, i.e. a tree, each of whose vertices is represented by a pseudoholomorphic sphere, with edges representing nodes - given by marked points on different spheres at which the maps satisfy a matching condition.
We will see that sphere bubbling does not contribute to the boundary stratification of these moduli spaces, so that the boundary stratification and thus the algebraic structure arising from these moduli spaces is induced by Floer breaking and disk bubbling. (On the other hand, sphere bubbling will be the only source of nontrivial isotropy.) The boundary strata arising from Floer breaking are fiber products of other moduli spaces of pseudoholomorphic polygons over finite sets of Lagrangian intersection points, which indicates an algebraic composition in this finitely generated Floer chain complex.
Disk bubbling, on the other hand, in the present setting yields boundary strata that are fiber products over the Lagrangian submanifold specified by the boundary condition, which is problematic for a combination of algebra and regularity reasons.
The corresponding algebraic composition requires a push-pull construction on some space of chains, currents, or differential forms on the Lagrangian. However, such constructions require transversality of the chains to the evaluation maps from the regularized moduli spaces, so that a rigorous construction of the 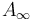 -structure in this setting - as in the approach by Fukaya et al - requires a complicated infinite iteration.
-structure in this setting - as in the approach by Fukaya et al - requires a complicated infinite iteration.
We will resolve this issue as in [J.Li thesis] by following another earlier proposal by Fukaya-Oh to allow disks to flow apart along a Morse trajectory, thus yielding disk trees which are constructed next - still ignoring sphere bubbling.
Pseudoholomorphic disk trees for a fixed Lagrangian
If the Lagrangians are all the same,  , then our construction is based on pseudoholomorphic disks
, then our construction is based on pseudoholomorphic disks
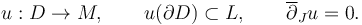
Such disks (modulo reparametrization by biholomorphisms of the disk) also arise from Gromov-compactifying other moduli spaces of pseudoholomorphic curves in which energy concentrates at a boundary point.
To capture this bubbling algebraically, we work throughout with the Morse function 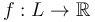 chosen in the setup of the morphism space
chosen in the setup of the morphism space 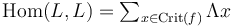 . We also choose a metric on
. We also choose a metric on 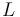 so that the gradient vector field
so that the gradient vector field 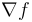 satisfies the Morse-Smale conditions and an additional technical assumption in [1] which guarantees a natural smooth manifold-with-boundary-and-corners structure on the compactified Morse trajectory spaces
satisfies the Morse-Smale conditions and an additional technical assumption in [1] which guarantees a natural smooth manifold-with-boundary-and-corners structure on the compactified Morse trajectory spaces
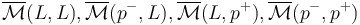 for
for 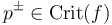 .
This smooth structure is essentially induced by the requirement that the evaluation maps at positive and negative ends
.
This smooth structure is essentially induced by the requirement that the evaluation maps at positive and negative ends 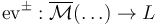 are smooth.
With that data and the fixed almost complex structure
are smooth.
With that data and the fixed almost complex structure  we can construct the moduli spaces of pseudoholomorphic disk trees for any tuple
we can construct the moduli spaces of pseudoholomorphic disk trees for any tuple 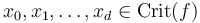 as in JL:
as in JL:
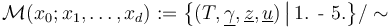
where
1. 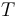 is an ordered tree with sets of vertices
is an ordered tree with sets of vertices 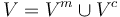 and edges
and edges 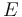 ,
,
equipped with orientations towards the root, orderings of incoming edges, and a partition into main and critical (leaf and root) vertices as follows:
- The edges
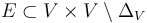 are oriented towards the root vertex
are oriented towards the root vertex 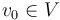 of the tree, i.e. for
of the tree, i.e. for 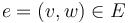 the outgoing vertex
the outgoing vertex 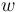 is still connected to the root after removing
is still connected to the root after removing 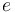 . Thus each vertex
. Thus each vertex 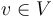 has a unique outgoing edge
has a unique outgoing edge 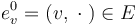 (except for the root vertex which has no outgoing edge) and a (possibly empty) set of incoming edges
(except for the root vertex which has no outgoing edge) and a (possibly empty) set of incoming edges 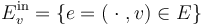 . Moreover, the set of incoming edges is ordered,
. Moreover, the set of incoming edges is ordered, 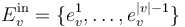 with
with 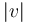 denoting the valence - number of attached edges - of
denoting the valence - number of attached edges - of 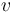 .
. - The set of vertices is partitioned
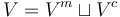 into the sets of main vertices
into the sets of main vertices  and the set of critical vertices
and the set of critical vertices 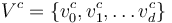 . The latter is ordered to start with the root
. The latter is ordered to start with the root 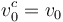 , which is required to have a single edge
, which is required to have a single edge 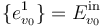 , and then contains d leaves
, and then contains d leaves 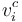 of the tree (i.e. with
of the tree (i.e. with 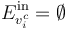 ), with order induced by the orientation and order of the edges (with the root being the minimal vertex).
), with order induced by the orientation and order of the edges (with the root being the minimal vertex).
2. 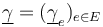 is a tuple of generalized Morse trajectories for each edge
is a tuple of generalized Morse trajectories for each edge
in the following compactified Morse trajectory spaces:
-
 for any edge
for any edge 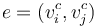 between critical vertices;
between critical vertices; 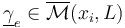 for any edge
for any edge 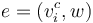 from a critical vertex
from a critical vertex 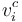 to a main vertex
to a main vertex  ;
;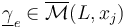 for any edge
for any edge 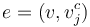 from a main vertex
from a main vertex  to a critical vertex
to a critical vertex 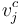 ;
;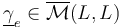 for any edge
for any edge 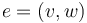 between main vertices
between main vertices  .
.
3. 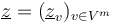 is a tuple of boundary marked points for each main vertex
is a tuple of boundary marked points for each main vertex
that correspond to the edges of 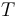 and are ordered counter-clockwise as follows:
and are ordered counter-clockwise as follows:
- For each main vertex
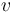 there are
there are 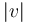 pairwise disjoint marked points
pairwise disjoint marked points 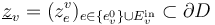 on the boundary of a disk.
on the boundary of a disk. - The order
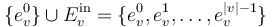 of the edges corresponds to a counter-clockwise order of the marked points
of the edges corresponds to a counter-clockwise order of the marked points 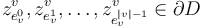 .
. - The marked points can also be denoted as
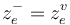 and
and 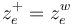 by the edges
by the edges 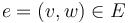 for which
for which  or
or  .
.
4. 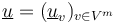 is a tuple of pseudoholomorphic disks for each main vertex,
is a tuple of pseudoholomorphic disks for each main vertex,
that is each  is labeled by a smooth map
is labeled by a smooth map 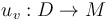 satisfying
Cauchy-Riemann equation, Lagrangian boundary condition, finite energy, and matching conditions as follows:
satisfying
Cauchy-Riemann equation, Lagrangian boundary condition, finite energy, and matching conditions as follows:
- The Cauchy-Riemann equation is
 .
. - The Lagrangian boundary condition is
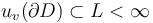 .
. - The finite energy condition is
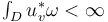 .
. - The pseudholomorphic disks can also be indexed as
 and
and 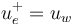 by the edges
by the edges 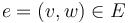 for which
for which  or
or  . In that notation, they satisfy the matching conditions with the generalized Morse trajectories
. In that notation, they satisfy the matching conditions with the generalized Morse trajectories 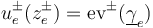 whenever
whenever 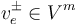 .
.
5. The disk tree is stable
in the sense that
any main vertex  whose disk has zero energy
whose disk has zero energy 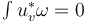 (which is equivalent to
(which is equivalent to 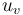 being constant) has valence
being constant) has valence 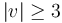 .
.
Finally, two pseudoholomorphic disk trees are equivalent  if
if
there is a tree isomorphism 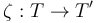 and a tuple of disk biholomorphisms
and a tuple of disk biholomorphisms 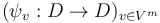 which preserve the tree, Morse trajectories, marked points, and pseudoholomorphic curves in the sense that
which preserve the tree, Morse trajectories, marked points, and pseudoholomorphic curves in the sense that
-
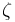 preserves the tree structure and order of edges;
preserves the tree structure and order of edges; 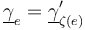 for every
for every  ;
;-
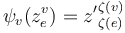 for every
for every  and adjacent edge
and adjacent edge  ;
; - the pseudoholomorphic disks are related by reparametrization,
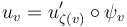 for every
for every  .
.
The domains of the disk trees are never stable for 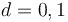 , but need to be studied to construct the
differential
, but need to be studied to construct the
differential 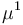 on the Floer chain complex and the curvature term
on the Floer chain complex and the curvature term 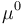 that may obstruct
that may obstruct 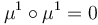 .
For
.
For 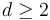 the domains of the disk trees represent elements in a Deligne-Mumford space as follows:
the domains of the disk trees represent elements in a Deligne-Mumford space as follows:
Any equivalence class of disk trees ![[(T,\underline {\gamma },\underline {z},\underline {u})]](/images/math/3/8/3/383800ad42d3c5072e12f54b0a6fe3f9.png) induces a domain tree
induces a domain tree ![[(T',\underline {\ell },\underline {z})]](/images/math/d/4/1/d41dd318d5fd7a04cf8aa75ae103c19e.png)
by forgetting the Morse trajectories and pseudoholomorphic maps as follows:
- The tree
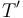 is obtained from
is obtained from 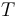 by replacing critical vertices and their outgoing edges by incoming semi-infinite edges of the new tree
by replacing critical vertices and their outgoing edges by incoming semi-infinite edges of the new tree 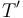 . We also replace the critical root vertex and its incoming edge by an outgoing semi-infinite edge of the new tree
. We also replace the critical root vertex and its incoming edge by an outgoing semi-infinite edge of the new tree 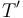 . The new tree
. The new tree 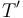 retains the orientations of edges and inherits an order of the edges from
retains the orientations of edges and inherits an order of the edges from 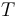 . Its root is the unique main vertex from which there was an edge to the critical root vertex in
. Its root is the unique main vertex from which there was an edge to the critical root vertex in 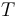 .
. - Every vertex
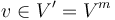 of
of 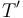 then represents a disk domain
then represents a disk domain 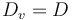 .
. - Every edge
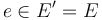 is labeled with the length
is labeled with the length ![\ell _{e}:=\ell (\underline {\gamma }_{e})\in [0,\infty ]](/images/math/5/c/0/5c00882bd14859421d79bd58c855a5d3.png) of the associated generalized Morse trajectory. For the semi-infinite edges, this length is automatically
of the associated generalized Morse trajectory. For the semi-infinite edges, this length is automatically 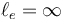 since the associated Morse trajectories are semi-infinite.
since the associated Morse trajectories are semi-infinite. - The domain for each vertex
 is marked by
is marked by 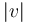 boundary points
boundary points 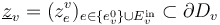 , ordered counter-clockwise.
, ordered counter-clockwise. - Two such trees are equivalent
![[(T,\underline {\ell },\underline {z})]\sim [(T',\underline {\ell }',\underline {z}')]](/images/math/b/a/2/ba206065fa9403003359115ed475c49e.png) if there is a tree isomorphism
if there is a tree isomorphism 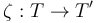 and a tuple of disk biholomorphisms
and a tuple of disk biholomorphisms 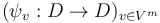 such that
such that 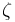 preserves the ordered tree structure and lengths
preserves the ordered tree structure and lengths 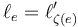 for every
for every  , and the marked points are preserved
, and the marked points are preserved 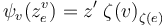 for every
for every  and adjacent
and adjacent  .
.
For 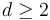 , such a domain tree is called stable if every vertex has valence
, such a domain tree is called stable if every vertex has valence 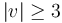 - i.e. there are at least three marked points on each disk
- i.e. there are at least three marked points on each disk  .
The domain trees for
.
The domain trees for 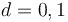 are never stable, but both cases need to be included in our moduli space constructions:
The differential
are never stable, but both cases need to be included in our moduli space constructions:
The differential 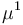 on the Floer chain complex
on the Floer chain complex 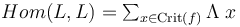 is constructed by counting the elements of
is constructed by counting the elements of 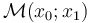 .
The curvature term
.
The curvature term 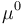 , which is constructed from moduli spaces
, which is constructed from moduli spaces 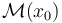 with no incoming critical points, serves to algebraically encode disk bubbling in any moduli space involving a Lagrangian boundary condition on
with no incoming critical points, serves to algebraically encode disk bubbling in any moduli space involving a Lagrangian boundary condition on 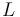 .
.
For 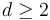 , while the above trees are not necessarily stable, they induce unique stable rooted metric ribbon trees
, while the above trees are not necessarily stable, they induce unique stable rooted metric ribbon trees  in the sense of [Def.2.7, MW], by forgetting the marked points, forgetting every leaf of valence 1 and its outgoing edge, and replacing every vertex
in the sense of [Def.2.7, MW], by forgetting the marked points, forgetting every leaf of valence 1 and its outgoing edge, and replacing every vertex 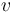 of valence 2 and its incoming and outgoing edges
of valence 2 and its incoming and outgoing edges 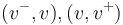 by a single edge
by a single edge 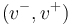 of length
of length 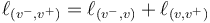 . The space of such stable rooted metric ribbon trees - where a tree containing an edge of length
. The space of such stable rooted metric ribbon trees - where a tree containing an edge of length  is identified with the tree in which this edge and its adjacent vertices are replaced by a single vertex - is another topological representation of the Deligne Mumford space
is identified with the tree in which this edge and its adjacent vertices are replaced by a single vertex - is another topological representation of the Deligne Mumford space  , as discussed in [BV].
Its boundary strata are given by trees with interior edges of length
, as discussed in [BV].
Its boundary strata are given by trees with interior edges of length 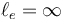 .
.
We now expect the boundary stratification of the moduli spaces of disk trees 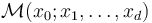 - if/once regular - to arise exclusively from breaking of the Morse trajectories representing edges of the disk trees. This is made rigorous in [J.Li thesis] under the assumption that the almost complex structure
- if/once regular - to arise exclusively from breaking of the Morse trajectories representing edges of the disk trees. This is made rigorous in [J.Li thesis] under the assumption that the almost complex structure  can be chosen such that there exist no nonconstant
can be chosen such that there exist no nonconstant  -holomorphic spheres in the symplectic manifold
-holomorphic spheres in the symplectic manifold 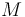 .
In that special case, all isotropy groups are trivial by [Prop.2.5, J.Li thesis]; that is any equivalence between a disk tree and itself,
.
In that special case, all isotropy groups are trivial by [Prop.2.5, J.Li thesis]; that is any equivalence between a disk tree and itself,
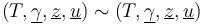 , is given by the trivial tree isomorphism
, is given by the trivial tree isomorphism 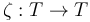 , and the only disk biholomorphisms
, and the only disk biholomorphisms 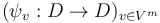 which preserve the marked points and pseudoholomorphic disk maps are the identity maps
which preserve the marked points and pseudoholomorphic disk maps are the identity maps 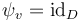 .
In this case, the moduli spaces of disk trees
.
In this case, the moduli spaces of disk trees 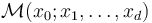 are moreover Gromov-compact since sphere bubbling is ruled out and disk bubbling is captured by edges labeled with constant, zero length, Morse trajectories.
are moreover Gromov-compact since sphere bubbling is ruled out and disk bubbling is captured by edges labeled with constant, zero length, Morse trajectories.
In general, we will Gromov-compactify 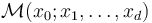 in the general construction below by allowing for sphere bubble trees (which we formalize next) to develop at any point of the disk and polygon domains. These will also be a source of generally nontrivial isotropy.
in the general construction below by allowing for sphere bubble trees (which we formalize next) to develop at any point of the disk and polygon domains. These will also be a source of generally nontrivial isotropy.
Sphere bubble trees
The sphere bubble trees that are relevant to the compactification of the moduli spaces of pseudoholomorphic polygons are genus zero stable maps with one marked point, as described in e.g. [Chapter 5, McDuff-Salamon].
For a fixed almost complex structure  , we can use the combinatorial simplification of working with a single marked point to construct the moduli space of sphere bubble trees as
, we can use the combinatorial simplification of working with a single marked point to construct the moduli space of sphere bubble trees as
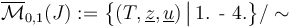
where
1. 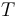 is a tree with sets of vertices
is a tree with sets of vertices 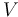 and edges
and edges 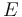 , and a distinguished root vertex
, and a distinguished root vertex 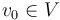 , which we use to orient all edges towards the root.
, which we use to orient all edges towards the root.
2. 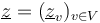 is a tuple of marked points on the spherical domains
is a tuple of marked points on the spherical domains 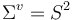 ,
,
indexed by the edges of 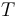 , and including a special root marked point as follows:
, and including a special root marked point as follows:
- For each vertex
 the tuple of mutually disjoint marked points
the tuple of mutually disjoint marked points 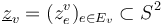 is indexed by the edges
is indexed by the edges  adjacent to
adjacent to 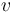 .
. - For the root vertex
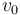 the tuple of mutually disjoint marked points
the tuple of mutually disjoint marked points 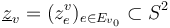 is also indexed by the edges adjacent to
is also indexed by the edges adjacent to 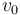 , but is also required to be disjoint from the fixed marked point
, but is also required to be disjoint from the fixed marked point 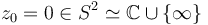 .
. - The marked points, except for
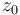 , can also be denoted as
, can also be denoted as 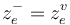 and
and 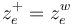 by the edges
by the edges 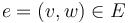 .
.
3.  is a tuple of pseudoholomorphic spheres for each vertex,
is a tuple of pseudoholomorphic spheres for each vertex,
that is each 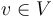 is labeled by a smooth map
is labeled by a smooth map 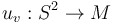 satisfying
Cauchy-Riemann equation, finite energy, and matching conditions as follows:
satisfying
Cauchy-Riemann equation, finite energy, and matching conditions as follows:
- The Cauchy-Riemann equation is
 .
. - The finite energy condition is
 .
. - The matching conditions are
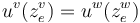 for each edge
for each edge 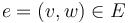 .
.
4. The sphere bubble tree is stable
in the sense that
any vertex 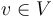 whose map has zero energy
whose map has zero energy 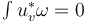 (which is equivalent to
(which is equivalent to 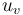 being constant) has valence
being constant) has valence 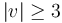 . Here the marked point
. Here the marked point 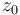 counts as one towards the valence
counts as one towards the valence 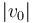 of the root vertex; in other words the root vertex can be constant with just two adjacent edges.
of the root vertex; in other words the root vertex can be constant with just two adjacent edges.
Finally, two sphere bubble trees are equivalent  if
if
there is a tree isomorphism 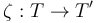 and a tuple of sphere biholomorphisms
and a tuple of sphere biholomorphisms 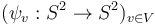 which preserve the tree, marked points, and pseudoholomorphic curves in the sense that
which preserve the tree, marked points, and pseudoholomorphic curves in the sense that
-
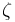 preserves the tree structure, in particular maps the root
preserves the tree structure, in particular maps the root 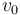 to the root
to the root 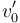 ;
; -
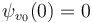 and
and 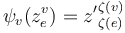 for every
for every 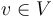 and adjacent edge
and adjacent edge  ;
; - the pseudoholomorphic spheres are related by reparametrization,
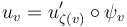 for every
for every 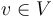 .
.
To attach such sphere bubble trees to the generalized pseudoholomorphic polygons below, we will use the evaluation map (which is well defined independent of the choice of representative)
![{\text{ev}}_{0}\,:\;\overline {\mathcal {M}}_{{0,1}}(J)\;\to \;M,\qquad {\bigl [}T,\underline {z},\underline {u}{\bigr ]}\;\mapsto \;u_{{v_{0}}}(0).](/images/math/b/2/c/b2c4358ba5993251dac8955347d97d44.png)
We will moreover make use of the symplectic area function
![\omega \,:\;\overline {\mathcal {M}}_{{0,1}}(J)\;\to \;\mathbb{R} \qquad {\bigl [}T,\underline {z},\underline {u}{\bigr ]}\;\mapsto \;\textstyle \sum _{{v\in V}}\int _{{S^{2}}}u_{v}^{*}\omega \;=\;\langle [\omega ],\sum _{{v\in V}}(u_{v})_{*}[S^{2}]\rangle ,](/images/math/5/8/1/581b3705d6a0eabc7760a3ebc377e6e8.png)
which only depends on the total homology class of the sphere bubble tree ![\beta ={\bigl [}T,\underline {z},\underline {u}{\bigr ]}](/images/math/f/2/d/f2d5b0c9aced1e0f3001e251fbe0e2e7.png) ,
,
![\textstyle [\beta ]:=\sum _{{v\in V}}(u_{v})_{*}[S^{2}]\;\in \;H_{2}(M).](/images/math/7/4/0/740f41a1395a2337b571acd9f6086e77.png)
General moduli space of pseudoholomorphic polygons
For the construction of a general 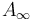 -composition map we are given
-composition map we are given 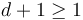 Lagrangians
Lagrangians 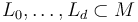 and a fixed autonomous Hamiltonian function
and a fixed autonomous Hamiltonian function 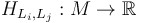 for each pair
for each pair 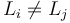 whose time-1 flow provides transverse intersections
whose time-1 flow provides transverse intersections  .
To simplify notation for consecutive Lagrangians in the list, we index it cyclically by
.
To simplify notation for consecutive Lagrangians in the list, we index it cyclically by 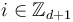 and abbreviate
and abbreviate 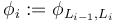 so that we have
so that we have 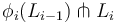 whenever
whenever 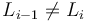 , and in particular
, and in particular 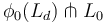 unless
unless 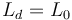 .
Now, given generators
.
Now, given generators 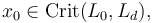

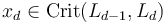 of these morphism spaces, we construct the Gromov-compactified moduli space of generalized pseudoholomorphic polygons by combining the two special cases above with sphere bubble trees,
of these morphism spaces, we construct the Gromov-compactified moduli space of generalized pseudoholomorphic polygons by combining the two special cases above with sphere bubble trees,
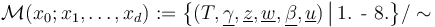
where
1. 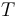 is an ordered tree with sets of vertices
is an ordered tree with sets of vertices 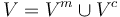 and edges
and edges 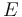 ,
,
equipped with orientations towards the root, orderings of incoming edges, and a partition into main and critical (leaf and root) vertices as follows:
- The edges
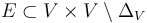 are oriented towards the root vertex
are oriented towards the root vertex 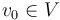 of the tree, so that each vertex
of the tree, so that each vertex 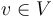 has a unique outgoing edge
has a unique outgoing edge 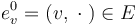 (except for the root vertex which has no outgoing edge) and a (possibly empty) set of incoming edges
(except for the root vertex which has no outgoing edge) and a (possibly empty) set of incoming edges 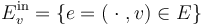 .
. - The set of incoming edges is ordered,
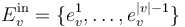 . This induces a cyclic order on the set of all edges
. This induces a cyclic order on the set of all edges 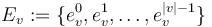 adjacent to
adjacent to 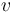 , by setting
, by setting 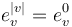 , and we will denote consecutive edges in this order by
, and we will denote consecutive edges in this order by 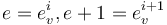 . In particular this yields
. In particular this yields 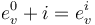 .
. - The set of vertices is partitioned
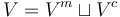 into the sets of main vertices
into the sets of main vertices  and critical vertices
and critical vertices 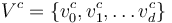 . The latter is ordered to start with the root
. The latter is ordered to start with the root 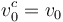 and then contains d leaves
and then contains d leaves 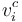 of the tree, with order induced by the orientation and order of the edges.
of the tree, with order induced by the orientation and order of the edges. - The root vertex
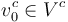 has a single edge
has a single edge 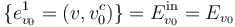 , and this attaches to a main vertex
, and this attaches to a main vertex  except for one special case: For
except for one special case: For 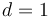 and
and 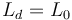 we allow the tree with a single edge
we allow the tree with a single edge 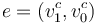 between its two critical vertices
between its two critical vertices 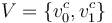 .
.
2. The tree structure induces tuples of Lagrangians 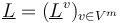
that label the boundary components of domains in overall counter-clockwise order  as follows:
as follows:
- For each main vertex
 the Lagrangian label
the Lagrangian label 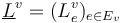 is a cyclic sequence of Lagrangians
is a cyclic sequence of Lagrangians 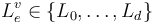 indexed by the adjacent edges
indexed by the adjacent edges 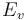 (which will become the boundary condition on
(which will become the boundary condition on 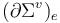 ).
). - For each edge
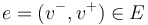 the Lagrangian labels satisfy a matching condition as follows:
the Lagrangian labels satisfy a matching condition as follows:
- The edge from a critical leaf
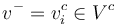 requires
requires 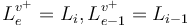 .
. - The edge to the critical root
 requires
requires 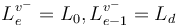 .
. - Any edge between main vertices
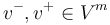 requires
requires 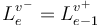 and
and 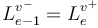 .
. - Since
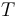 has no further leaves, this determines the Lagrangian labels uniquely.
has no further leaves, this determines the Lagrangian labels uniquely.
- The edge from a critical leaf
3. 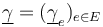 is a tuple of generalized Morse trajectories
is a tuple of generalized Morse trajectories
in the following compactified Morse trajectory spaces:
- Any edge
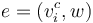 from a critical leaf
from a critical leaf 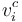 to a main vertex
to a main vertex  is labeled by a half-infinite Morse trajectory
is labeled by a half-infinite Morse trajectory 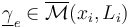 if
if 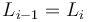 , resp. by the constant
, resp. by the constant 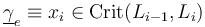 in the discrete space
in the discrete space 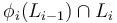 if
if 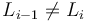 .
. - If the edge to the root
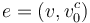 attaches to a main vertex
attaches to a main vertex  then it is labeled by a half-infinite Morse trajectory
then it is labeled by a half-infinite Morse trajectory 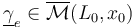 if
if 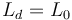 , resp. by the constant
, resp. by the constant 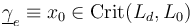 in the discrete space
in the discrete space 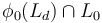 if
if  .
. - An edge
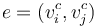 between critical vertices is labeled by an infinite Morse trajectory
between critical vertices is labeled by an infinite Morse trajectory  (this occurs only for
(this occurs only for 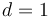 with
with 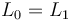 and the tree with one edge
and the tree with one edge 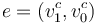 ).
). - Any edge
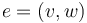 between main vertices
between main vertices  is labeled by a finite or infinite Morse trajectory
is labeled by a finite or infinite Morse trajectory 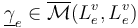 in case
in case 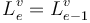 , resp. by a constant
, resp. by a constant 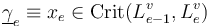 in the discrete space
in the discrete space 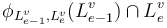 in case
in case 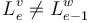 . (Recall the matching condition
. (Recall the matching condition 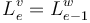 and
and 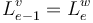 from 2.)
from 2.)
4. 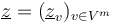 is a tuple of boundary points
is a tuple of boundary points
that correspond to the edges of 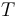 , are ordered counter-clockwise, and associate complex domains
, are ordered counter-clockwise, and associate complex domains 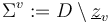 to the vertices as follows:
to the vertices as follows:
- For each main vertex
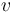 there are
there are 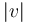 pairwise disjoint marked points
pairwise disjoint marked points 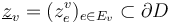 on the boundary of a disk.
on the boundary of a disk. - The order
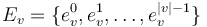 of the edges corresponds to a counter-clockwise order of the marked points
of the edges corresponds to a counter-clockwise order of the marked points 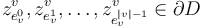 .
. - The marked points can also be denoted as
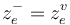 and
and 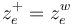 by the edges
by the edges 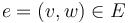 for which
for which  or
or 
- To each main vertex
 we associate the punctured disk
we associate the punctured disk 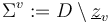 . Then the marked points
. Then the marked points 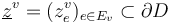 partition the boundary into
partition the boundary into 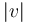 connected components
connected components 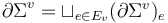 such that the closure of each component
such that the closure of each component 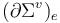 contains the marked points
contains the marked points 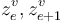 .
.
5. 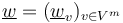 is a tuple of sphere bubble tree attaching points for each main vertex
is a tuple of sphere bubble tree attaching points for each main vertex  , given by an unordered subset
, given by an unordered subset 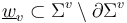 of the interior of the domain.
of the interior of the domain.
6. 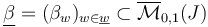 is a tuple of sphere bubble trees
is a tuple of sphere bubble trees 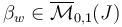 indexed by the disjoint union
indexed by the disjoint union 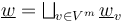 of sphere bubble tree attaching points.
of sphere bubble tree attaching points.
7. 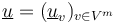 is a tuple of pseudoholomorphic maps for each main vertex,
is a tuple of pseudoholomorphic maps for each main vertex,
that is each  is labeled by a smooth map
is labeled by a smooth map 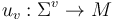 satisfying Cauchy-Riemann equation, Lagrangian boundary conditions, finite energy, and matching conditions as follows:
satisfying Cauchy-Riemann equation, Lagrangian boundary conditions, finite energy, and matching conditions as follows:
- The Cauchy-Riemann equation is

Here  is a vector-field-valued 1-form on
is a vector-field-valued 1-form on 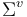 that is chosen compatibly with the fixed Hamiltonian perturbations as follows:
that is chosen compatibly with the fixed Hamiltonian perturbations as follows:
On the thin part ![\iota _{e}^{v}:[0,\infty )\times [0,1]\hookrightarrow \Sigma ^{v}](/images/math/d/1/1/d1100c1e7510c1014aac509f6b8fd6de.png) near each puncture
near each puncture 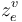 we have
we have  .
.
In particular, this convention together with our symmetric choice of Hamiltonian perturbations  forces the vector-field-valued 1-form on
forces the vector-field-valued 1-form on ![\Sigma ^{v}\simeq \mathbb{R} \times [0,1]](/images/math/5/6/a/56a3486ce4c38533929719c2fae3d318.png) in case
in case 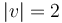 to be
to be 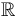 -invariant,
-invariant,  if
if 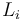 are the Lagrangian labels for the boundary components
are the Lagrangian labels for the boundary components 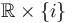 .
.
Here and in the following we denote  in case
in case  , so that
, so that  in case
in case 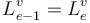 .
.
- The Lagrangian boundary conditions are
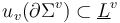 ; more precisely this requires
; more precisely this requires  for each adjacent edge
for each adjacent edge  .
. - The finite energy condition is
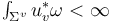 .
. - The matching conditions for sphere bubble trees are
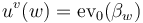 for each main vertex
for each main vertex  and sphere bubble tree attaching point
and sphere bubble tree attaching point 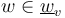 .
. - Finite energy together with the (perturbed) Cauchy-Riemann equation implies uniform convergence of
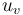 near each puncture
near each puncture 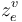 , and the limits are required to satisfy the following matching conditions:
, and the limits are required to satisfy the following matching conditions:
- For edges
 whose Lagrangian boundary conditions
whose Lagrangian boundary conditions 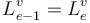 agree, the map
agree, the map 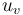 extends smoothly to the puncture
extends smoothly to the puncture 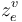 , and its value is required to match with the evaluation of the Morse trajectory
, and its value is required to match with the evaluation of the Morse trajectory 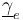 associated to the edge
associated to the edge 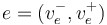 , that is
, that is 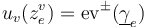 for
for 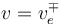 .
. - For edges
 with different Lagrangian boundary conditions
with different Lagrangian boundary conditions  , the map
, the map ![u_{e}^{v}:=(\iota _{e}^{v})^{*}u_{v}:(-\infty ,0)\times [0,1]\to M](/images/math/6/3/7/63792330def3bf1b30267f5afb4d519f.png) has a uniform limit
has a uniform limit 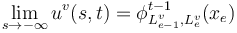 for some
for some 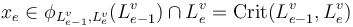 , and this limit intersection point is required to match with the value of the constant 'Morse trajectory'
, and this limit intersection point is required to match with the value of the constant 'Morse trajectory' 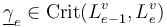 associated to the edge
associated to the edge 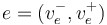 , that is
, that is  .
.
- For edges
8. The generalized pseudoholomorphic polygon is stable
in the sense that
any main vertex  whose map has zero energy
whose map has zero energy 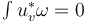 has enough special points to have trivial isotropy, that is the number of boundary marked points
has enough special points to have trivial isotropy, that is the number of boundary marked points 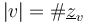 plus twice the number of interior marked points
plus twice the number of interior marked points 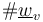 is at least 3.
is at least 3.
Finally, two generalized pseudoholomorphic polygons are equivalent 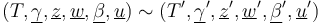 if
if
there is a tree isomorphism 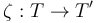 and a tuple of disk biholomorphisms
and a tuple of disk biholomorphisms 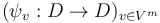 which preserve the tree, Morse trajectories, marked points, and pseudoholomorphic curves in the sense that
which preserve the tree, Morse trajectories, marked points, and pseudoholomorphic curves in the sense that
-
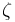 preserves the tree structure and order of edges;
preserves the tree structure and order of edges; 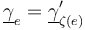 for every
for every  ;
;-
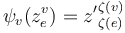 for every
for every  and adjacent edge
and adjacent edge  ;
; -
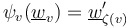 for every
for every  ;
; -
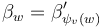 for every
for every  and
and 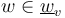 ;
; - the pseudoholomorphic maps are related by reparametrization,
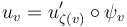 for every
for every  .
.
Warning: Our directional conventions differ somewhat from [Seidel book] and [J.Li thesis] as follows:
Unlike both references, we orient edges towards the root, in order to obtain a more natural interpretation of the leaves as incoming vertices as in [J.Li thesis], but unlike [Seidel book] which uses the language of 1 incoming striplike end and 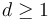 outgoing striplike ends.
Since we also insist on ordering the marked points counter-clockwise on the boundary of the disk, we then have to work with positive striplike ends
outgoing striplike ends.
Since we also insist on ordering the marked points counter-clockwise on the boundary of the disk, we then have to work with positive striplike ends ![[0,\infty )\times [0,1]\hookrightarrow \Sigma ^{v}](/images/math/b/e/c/bec1bfe842c6b1916c804663467dcec7.png) near each marked point
near each marked point 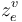 for an incoming edge
for an incoming edge 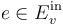 to make sure that the boundary components are labeled in order:
to make sure that the boundary components are labeled in order: 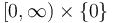 with
with 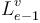 , and
, and 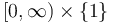 with
with 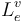 .
Analogously, a negative striplike end
.
Analogously, a negative striplike end ![(-\infty ,0]\times [0,1]\hookrightarrow \Sigma ^{v}](/images/math/c/7/3/c732b45e4625a7ffd19f60df1348fade.png) near the marked point
near the marked point 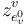 for the outgoing edge labels
for the outgoing edge labels 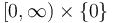 with
with 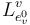 and
and 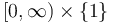 with
with 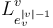 .
.
This amounts to working on Floer cohomology in the sense that for e.g. 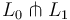 the output of the differential
the output of the differential  includes a sum over (amongst other more complicated trees) pseudoholomorphic strips
includes a sum over (amongst other more complicated trees) pseudoholomorphic strips ![b=[u:\mathbb{R} \times [0,1]\to M]](/images/math/d/9/6/d96a1d9a44f24bab4af54724bdfa170d.png) with fixed positive limit
with fixed positive limit 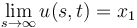 .
.
The symplectic area function on these moduli spaces is defined by
![\omega \,:\;\overline {\mathcal {M}}(x_{0};x_{1},\ldots ,x_{d})\;\to \;\mathbb{R} \qquad b={\bigl [}T,\underline {\gamma },\underline {z},\underline {w},\underline {\beta },\underline {u}{\bigr ]}\;\mapsto \;\omega (b):=\sum _{{v\in V^{m}}}\textstyle \int _{{\Sigma _{v}}}u_{v}^{*}\omega +\sum _{{\beta _{w}\in \underline {\beta }}}\omega (\beta _{w})\;=\;\langle [\omega ],[b]\rangle ,](/images/math/e/7/7/e7774c0212460b143bc43148a817c04c.png)
which - since  only depends on the total homology class of the generalized polygon
only depends on the total homology class of the generalized polygon
![[b]:=\sum _{{v\in V}}(\overline {u}_{v})_{*}[D]+\sum _{{\beta _{w}\in \underline {\beta }}}[\beta _{w}]\;\in \;H_{2}(M;L_{0}\cup L_{1}\ldots \cup L_{d}).](/images/math/3/2/1/321a107845d6cd857b754c4884454f7a.png)
Here 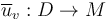 is defined by unique continuous continuation to the punctures
is defined by unique continuous continuation to the punctures 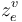 at which
at which 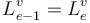 or
or 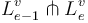 .
.
Differential Geometric TODO:
Worry about invariance of 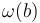 under continuous (or smooth) deformations with fixed limits ... should be fine, but how to say that? ... maybe not homologically?
under continuous (or smooth) deformations with fixed limits ... should be fine, but how to say that? ... maybe not homologically?